ramé-hart Newsletter

                  

September 2020
 

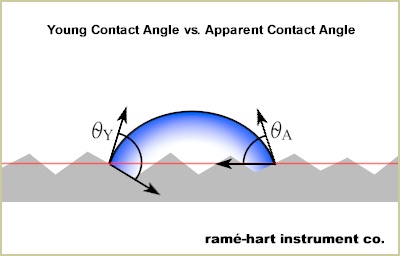
Now I've spent the second half of my life on a quest for an ideal surface. But sadly I report that I have not yet found one. Let's face it, the ideal surface is the Holy Grail of surface science. Many search but no one finds. 

This month, let's explore what exactly defines an ideal surface. But first, a quick review of Young's equation. The graphic below details the three forces that explain the contact angle of a liquid drop on a solid surface. This is the most basic and common mathematical description of wetting behavior.

However, in order to use Young's equation to understand wetting of a liquid sessile drop in equilibrium on a solid surface, it's assumed that the solid is an ideal surface. However, most if not all surfaces are not ideal. We call these non-ideal surfaces real surfaces. Here are the characteristics of the ephemeral ideal surface:

• There is no absorption of the liquid into the ideal surface.
   
• There is no swelling of the ideal surface by the liquid.
   
• The surface tension of the liquid phase remains constant. That is, there are no contaminants or other matter on the ideal surface which can affect the surface tension of the drop.
   
• The ideal surface is chemically and structurally homogeneous. Virtually all real surfaces exhibit some degree of heterogeneity.
   
• The ideal surface will be smoother than a fast-talking used car salesman. In technical terms, we're talking a surface roughness under 0.1 µm RMS. Most real surfaces fail this requirement as well.
   
• The ideal surface will not reorient as a result of the test liquid. Most polymer real surfaces are not immobile.
   
• The ideal surface will be rigid. Many real surfaces, including gels and elastomers, are not sufficiently rigid.

It should be noted that an ideal surface will have no contact angle hysteresis. (This point appears to be hotly debated but we'll save that discussion for a future newsletter.) We observe that real surfaces have some contact angle hysteresis and we conclude that the magnitude of the hysteresis is a reflection of the noncompliance to the rules of ideal surfaces as listed above.

When measuring contact angle on real surfaces, it's important to differentiate between the Young contact angle (θ_Y) and the apparent contact angle (θ_A). The difference can be significant.

When measuring a static contact angle using a goniometer, it's the apparent contact angle that's being measured. The angle is defined tangent to the drop and the surface baseline (red) as shown in the graphic above. The Young contact angle is measured between the drop tangent and the surface tangent at the microscopic level as illustrated above.

With an ideal surface, the Young contact angle (θ_Y) and the apparent contact angle (θ_A) are the same and cannot exceed about 120°. PTFE is an example of a material that approaches that maximal value when smooth and unstructured - that is, close to ideal. When an apparent contact angle of any material is measured in excess of 120°, it's not the underlying material alone that promotes the superhydrophobic behavior - rather, it's the surface roughness or topography.

Surface roughness amplifies wetting behavior. That is say that roughness result in better wetting for hydrophilic surfaces and less wetting for hydrophobic surfaces. Low contact angles get lower and high contact angles get higher. When the contact angle hysteresis is low, it signals that the surface is close to ideal - or, in other words, the surface has little roughness and limited heterogeneity. The exception to this rule are surfaces in the Cassie-Baxter state - they nearly always have low contact angle hysteresis but high levels of roughness.

So, if in your travels you find an ideal surface, be sure to measure the contact angle hysteresis. If it's near zero, you may have a winner. And if you can't find an ideal surface, you may want to start looking for an ideal mate. That will be you more joy in the long run anyway.

Bullets

• Young's Equation assumes an ideal surface - that is, one that is fully nondeformable, immobile, rigid, perfectly smooth and clean and 100% structurally and chemically homogenous.
• An ideal surface will have no contact angle hysteresis.
• Virtually all surfaces found in the real world are less than ideal. We call these real surfaces.
• With real surfaces, it's important to distinguish between the Young contact angle (θ_Y) which is relative to the structured surface at the microscopic level and the apparent contact angle (θ_A) which is measured relative to the overall surface using conventional goniometry methods.
• The maximum achievable contact angle on an ideal (or nearly) ideal surface is 120°. When the apparent contact angle is greater than 120°, it's attributable to surface roughness.
• Roughness amplifies wetting behavior. That is, it makes hydrophilic surfaces more so and hydrophobic surfaces more so.

Carl Clegg
Director of Sales
Phone 973-448-0305
www.ramehart.com
Contact us